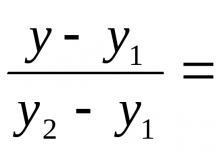Example 1

Reference: The following ways to denote a function are equivalent: In some tasks it is convenient to designate the function as "igrokom", and in some as "ff from x".

First, we find the derivative:

Example 2

Calculate the derivative of a function at a point

, , full function study and etc.

Example 3

Calculate the derivative of a function at a point. First, let's find the derivative:

Well, that's a completely different matter. Let's calculate the value of the derivative at the point:

In the event that you do not understand how the derivative was found, return to the first two lessons of the topic. If you have difficulty (misunderstanding) with the arctangent and its meanings, necessarily study the teaching material Graphs and properties of elementary functions- the most recent paragraph. Because there are still enough arctangents for the student age.

Example 4

Calculate the derivative of a function at a point.

Equation of a tangent to a graph of a function

To consolidate the previous section, consider the problem of finding the tangent to function graphics at this point. We met this task at school, and it is also found in the course of higher mathematics.

Let's consider a "demo" simplest example.

Write the equation of the tangent to the graph of the function at the point with the abscissa. I will immediately give a ready-made graphical solution to the problem (in practice, this is not necessary in most cases):

A strict definition of a tangent is given by definition of the derivative of a function, but for now we will master the technical part of the question. Surely almost everyone intuitively understands what a tangent is. If you explain "on the fingers", then the tangent to the graph of the function is straight which concerns the graph of the function in the only one point. In this case, all nearby points of the straight line are located as close as possible to the graph of the function.

As applied to our case: at, the tangent (standard notation) touches the graph of the function at a single point.

And our task is to find the equation of the line.

Derivative of a function at a point

How to find the derivative of a function at a point? Two obvious points of this assignment follow from the wording:

1) It is necessary to find the derivative.

2) It is necessary to calculate the value of the derivative at a given point.

Example 1

Calculate the derivative of a function at a point

Help: The following ways to denote a function are equivalent:


In some tasks it is convenient to designate the function as "igrokom", and in some as "ff from x".

First, we find the derivative:

I hope many have already got used to finding such derivatives orally.

At the second step, we calculate the value of the derivative at the point:

A small warm-up example for an independent solution:

Example 2

Calculate the derivative of a function at a point

Complete solution and answer at the end of the tutorial.

The need to find the derivative at a point arises in the following problems: construction of a tangent to the graph of a function (next paragraph), extremum function study , inflection of a graph function , full function study and etc.

But the task in question is found in tests and by itself. And, as a rule, in such cases, the function is given quite complex. In this regard, consider two more examples.

Example 3

Calculate the derivative of a function at the point.
First, let's find the derivative:

The derivative, in principle, has been found, and the required value can be substituted. But I don't really want to do it. The expression is very long, and the value of "x" is fractional. Therefore, we try to simplify our derivative as much as possible. In this case, let's try to bring the last three terms to a common denominator: at the point.

This is an example for a do-it-yourself solution.

How to find the value of the derivative of the function F (x) at the point Xo? How to solve this in general?

If the formula is given, then find the derivative and substitute X-zero instead of X. Calculate
If we are talking about b-8 USE, graph, then you need to find the tangent of the angle (acute or obtuse), which forms a tangent with the X-axis (using the mental construction of a right-angled triangle and determining the tangent of the angle)

Timur adilkhodzhaev

First, you need to decide on the sign. If the point x0 is in the lower part of the coordinate plane, then the sign in the answer will be a minus, and if it is higher, then +.
Secondly, you need to know what tanges is in a rectangular rectangle. And this is the ratio of the opposite side (leg) to the adjacent side (also leg). There are usually some black marks on the painting. From these marks you make a right-angled triangle and find tanges.

How to find the value of the derivative of the function f x at the point x0?

no specific question posed - 3 years ago

In general, in order to find the value of the derivative of any function with respect to some variable at any point, you need to differentiate the given function with respect to this variable. In your case, by the variable X. In the resulting expression, instead of X, put the value of x at the point for which you need to find the value of the derivative, i.e. in your case, substitute zero X and calculate the resulting expression.

Well, your desire to understand this issue, in my opinion, undoubtedly deserves +, which I put with a clear conscience.

This formulation of the problem of finding the derivative is often posed to fix the material on the geometric meaning of the derivative. A graph of a certain function is proposed, completely arbitrary and not given by an equation, and it is required to find the value of the derivative (not the derivative itself, note!) At the specified point X0. For this, a tangent line to a given function is constructed and the point of its intersection with the coordinate axes is found. Then the equation of this tangent line is drawn up in the form y = kx + b.

In this equation, the coefficient k and will be the value of the derivative. it remains only to find the value of the coefficient b. To do this, we find the value of y at x = o, let it be equal to 3 - this is the value of the coefficient b. We substitute the values ​​of X0 and Y0 into the original equation and find k - our value of the derivative at this point.

Problem B9 gives a graph of a function or derivative, from which you want to determine one of the following quantities:

1. The value of the derivative at some point x 0,
2. High or low points (extremum points),
3. The intervals of increasing and decreasing of the function (intervals of monotonicity).

The functions and derivatives presented in this problem are always continuous, which greatly simplifies the solution. Despite the fact that the task belongs to the section of mathematical analysis, it is quite within the power of even the weakest students, since no deep theoretical knowledge is required here.

There are simple and universal algorithms for finding the value of the derivative, extremum points and monotonicity intervals - all of them will be discussed below.

Carefully read the condition of problem B9 in order to avoid stupid mistakes: sometimes you come across rather lengthy texts, but there are not many important conditions that affect the course of the solution.

Calculating the value of the derivative. Two point method

If in the problem the graph of the function f (x) is given, tangent to this graph at some point x 0, and it is required to find the value of the derivative at this point, the following algorithm is applied:

1. Find two "adequate" points on the tangent graph: their coordinates must be integers. Let us denote these points by A (x 1; y 1) and B (x 2; y 2). Write out the coordinates correctly - this is a key point in the solution, and any mistake here leads to the wrong answer.
2. Knowing the coordinates, it is easy to calculate the increment of the argument Δx = x 2 - x 1 and the increment of the function Δy = y 2 - y 1.
3. Finally, we find the value of the derivative D = Δy / Δx. In other words, you need to divide the function increment by the argument increment - and this will be the answer.

Note once again: points A and B should be sought exactly on the tangent line, and not on the graph of the function f (x), as is often the case. The tangent line will necessarily contain at least two such points - otherwise the problem is not written correctly.

Consider points A (−3; 2) and B (−1; 6) and find the increments:
Δx = x 2 - x 1 = −1 - (−3) = 2; Δy = y 2 - y 1 = 6 - 2 = 4.

Find the value of the derivative: D = Δy / Δx = 4/2 = 2.

Task. The figure shows the graph of the function y = f (x) and the tangent to it at the point with the abscissa x 0. Find the value of the derivative of the function f (x) at the point x 0.

Consider points A (0; 3) and B (3; 0), find the increments:
Δx = x 2 - x 1 = 3 - 0 = 3; Δy = y 2 - y 1 = 0 - 3 = −3.

Now we find the value of the derivative: D = Δy / Δx = −3/3 = −1.

Task. The figure shows the graph of the function y = f (x) and the tangent to it at the point with the abscissa x 0. Find the value of the derivative of the function f (x) at the point x 0.

Consider points A (0; 2) and B (5; 2) and find the increments:
Δx = x 2 - x 1 = 5 - 0 = 5; Δy = y 2 - y 1 = 2 - 2 = 0.

It remains to find the value of the derivative: D = Δy / Δx = 0/5 = 0.

From the last example, we can formulate a rule: if the tangent is parallel to the OX axis, the derivative of the function at the point of tangency is zero. In this case, you don't even need to count anything - just look at the chart.

Calculating the maximum and minimum points

Sometimes, instead of a graph of a function, in Problem B9, a graph of the derivative is given and it is required to find the maximum or minimum point of the function. In this situation, the two-point method is useless, but there is another, even simpler algorithm. First, let's define the terminology:

1. A point x 0 is called a maximum point of the function f (x) if in some neighborhood of this point the following inequality holds: f (x 0) ≥ f (x).
2. A point x 0 is called a minimum point of the function f (x) if in some neighborhood of this point the following inequality holds: f (x 0) ≤ f (x).

In order to find the maximum and minimum points on the graph of the derivative, it is enough to perform the following steps:

1. Redraw the graph of the derivative, removing all unnecessary information. As practice shows, unnecessary data only interferes with the solution. Therefore, we mark the zeros of the derivative on the coordinate axis - that's all.
2. Find out the signs of the derivative at the intervals between zeros. If for some point x 0 it is known that f '(x 0) ≠ 0, then only two options are possible: f' (x 0) ≥ 0 or f '(x 0) ≤ 0. The sign of the derivative can be easily determined from the initial drawing: if the graph of the derivative lies above the OX axis, then f '(x) ≥ 0. And vice versa, if the graph of the derivative lies below the OX axis, then f' (x) ≤ 0.
3. Check the zeros and signs of the derivative again. Where the sign changes from minus to plus, there is a minimum point. Conversely, if the sign of the derivative changes from plus to minus, this is the maximum point. The counting is always carried out from left to right.

This scheme works only for continuous functions - there are no others in problem B9.

Task. The figure shows the graph of the derivative of the function f (x) defined on the segment [−5; 5]. Find the minimum point of the function f (x) on this segment.

Let's get rid of unnecessary information - we will leave only the borders [−5; 5] and zeros of the derivative x = −3 and x = 2.5. Also note the signs:

Obviously, at the point x = −3 the sign of the derivative changes from minus to plus. This is the minimum point.

Task. The figure shows the graph of the derivative of the function f (x), defined on the segment [−3; 7]. Find the maximum point of the function f (x) on this segment.

Let's redraw the graph, leaving only the boundaries [−3; 7] and the zeros of the derivative x = −1.7 and x = 5. Note the signs of the derivative on the resulting graph. We have:

Obviously, at the point x = 5 the sign of the derivative changes from plus to minus - this is the maximum point.

Task. The figure shows the graph of the derivative of the function f (x) defined on the segment [−6; 4]. Find the number of maximum points of the function f (x) that belong to the segment [−4; 3].

It follows from the problem statement that it is sufficient to consider only the part of the graph bounded by the segment [−4; 3]. Therefore, we build a new chart, on which we mark only the boundaries [−4; 3] and the zeros of the derivative inside it. Namely, points x = −3.5 and x = 2. We get:

This graph has only one maximum point x = 2. It is at this point that the sign of the derivative changes from plus to minus.

A quick note on points with non-integer coordinates. For example, in the last problem the point was considered x = −3.5, but you can just as well take x = −3.4. If the problem is formulated correctly, such changes should not affect the answer, since the points "without a definite abode" do not directly participate in solving the problem. Of course, this trick will not work with integer points.

Finding the intervals of increasing and decreasing functions

In such a problem, like the maximum and minimum points, it is proposed to find the regions in which the function itself increases or decreases from the derivative graph. First, let's define what is increasing and decreasing:

1. A function f (x) is called increasing on a segment if for any two points x 1 and x 2 from this segment the following statement is true: x 1 ≤ x 2 ⇒ f (x 1) ≤ f (x 2). In other words, the larger the argument value, the larger the function value.
2. A function f (x) is called decreasing on a segment if for any two points x 1 and x 2 from this segment the following statement is true: x 1 ≤ x 2 ⇒ f (x 1) ≥ f (x 2). Those. the larger the value of the argument, the smaller the value of the function.

Let us formulate sufficient conditions for increasing and decreasing:

1. For a continuous function f (x) to increase on a segment, it is sufficient that its derivative inside the segment is positive, i.e. f '(x) ≥ 0.
2. For a continuous function f (x) to decrease on a segment, it is sufficient that its derivative inside the segment is negative, i.e. f '(x) ≤ 0.

Let's accept these statements without proof. Thus, we get a scheme for finding the intervals of increase and decrease, which is in many ways similar to the algorithm for calculating extremum points:

1. Remove all unnecessary information. On the original plot of the derivative, we are primarily interested in the zeros of the function, so we will leave only them.
2. Note the signs of the derivative at the intervals between zeros. Where f ’(x) ≥ 0, the function increases, and where f’ (x) ≤ 0, decreases. If the problem has restrictions on the variable x, we additionally mark them on the new graph.
3. Now that we know the behavior of the function and the constraint, it remains to calculate the value required in the problem.

Task. The figure shows the graph of the derivative of the function f (x), defined on the segment [−3; 7.5]. Find the intervals of decrease of the function f (x). In your answer, indicate the sum of the integers included in these intervals.

As usual, redraw the graph and mark the boundaries [−3; 7.5], as well as the zeros of the derivative x = −1.5 and x = 5.3. Then we mark the signs of the derivative. We have:

Since the derivative is negative on the interval (- 1.5), this is the interval of decreasing function. It remains to sum up all the integers that are within this interval:
−1 + 0 + 1 + 2 + 3 + 4 + 5 = 14.

Task. The figure shows the graph of the derivative of the function f (x), defined on the segment [−10; 4]. Find the intervals of increase of the function f (x). In the answer, indicate the length of the longest of them.

Let's get rid of unnecessary information. Leave only the borders [−10; 4] and the zeros of the derivative, which this time turned out to be four: x = −8, x = −6, x = −3 and x = 2. Note the signs of the derivative and get the following picture:

We are interested in the intervals of increasing the function, i.e. such, where f '(x) ≥ 0. There are two such intervals on the graph: (−8; −6) and (−3; 2). Let's calculate their lengths:
l 1 = - 6 - (−8) = 2;
l 2 = 2 - (−3) = 5.

Since it is required to find the length of the largest of the intervals, in the answer we write down the value l 2 = 5.